Russia Tsunami on Mar. 25, 2020  


 

We have simulated the tsunami generated from the Russia earthquake (48.986°N, 157.693°E, depth = 56.7 km, M = 7.5 at 02:49:21 UTC according to USGS) on March 25, 2020 (Fig. 1). The assumed tsunami source is located including the epicenter (Fig. 1). The fault length and width are 40 km × 20 km. The focal mechanism is strike:204º, dip:48º, slip:89º from the USGS's W-phase moment tensor solution (NP1). The top depth of the fault was assumed to 40 km. The average slip on the fault is 4 m. The seismic moment is 2.24 x 10**20 Nm (Mw = 7.5) assuming the rigidity of 7 x 10**10 N/m**2. As the initial condition for tsunami, static deformation of the seafloor is calculated for a rectangular fault model [Okada, 1985] using the source model. The used bathymetry data is the 15 arc-second grid data from GEBCO_2019, which was resampled to 2 arc-minute grid data. To calculate tsunami propagation, the linear shallow-water, or long-wave, equations were numerically solved by using a finite-difference method [Satake, 1995]. We applied the phase correction method [Watada et al., 2014] to the calculated tsunami waveforms. We have downloaded the DART data from NOAA's web site, and compared the simulated tsunami waveforms and the observed ones (Fig. 2). We can see the tsunami propagation in the Pacific ocean (Fig. 3).


 

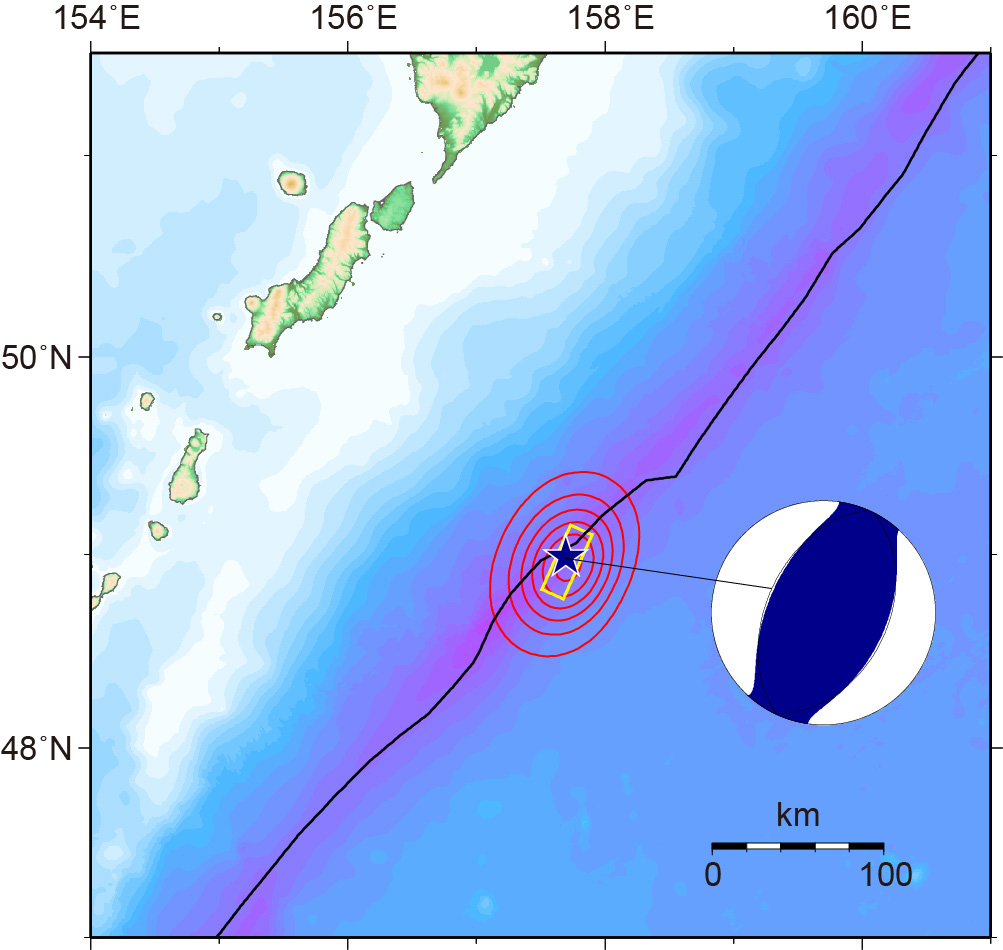
Fig.1 Tsunami Source Model

Fig.1 Tsunami Source Model
 The red lines indicate uplift with the contour interval of 0.05 m. The focal mechanism determined by USGS is also shown.


 

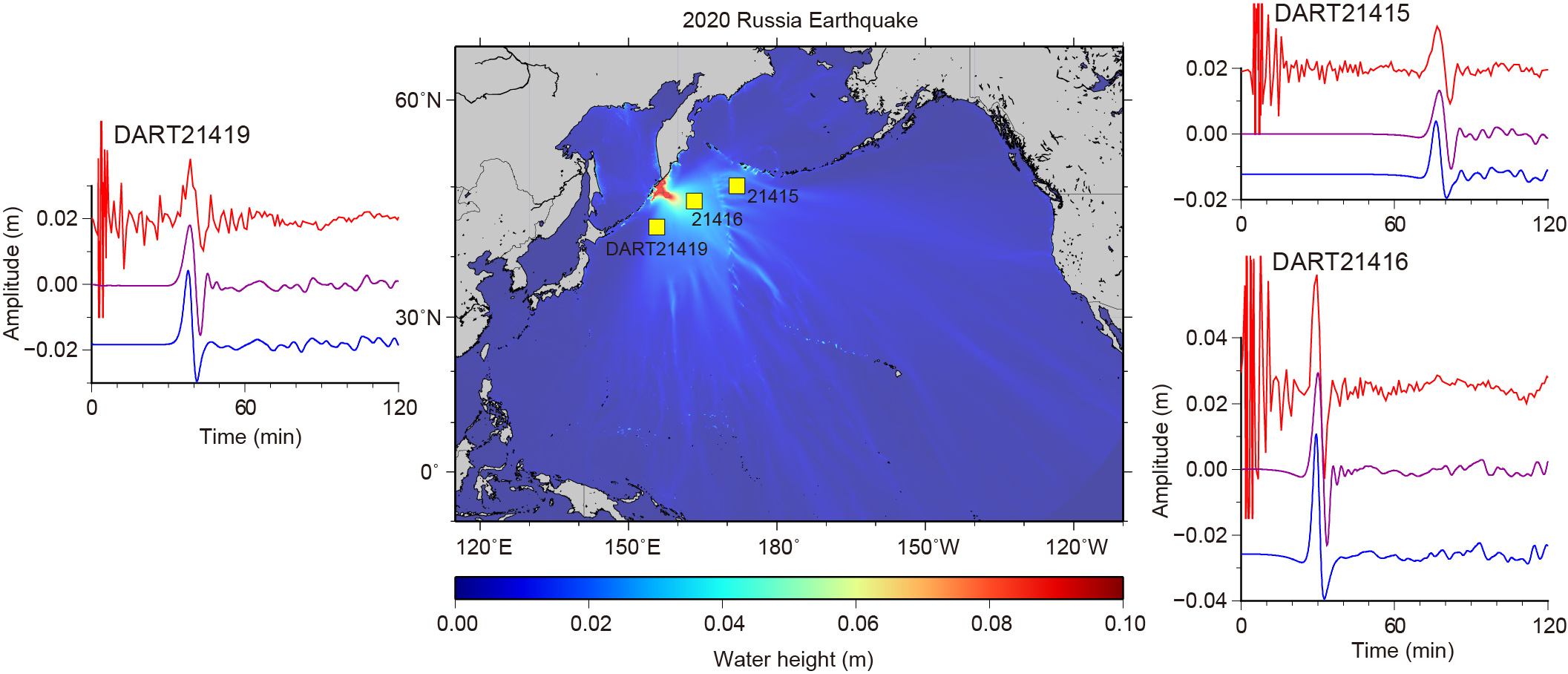
Fig.2 Maximum Height of Tsunami

Fig.2 Maximum Height of Simulated Tsunami and Tsunami Waveforms
 Solid lines in red and blue indicate the observed tsunami waveforms and calculated ones, respectively.
Purple lines show the synthetic tsunami waveforms by applying the phase correction method [Watada et al., 2014].


 


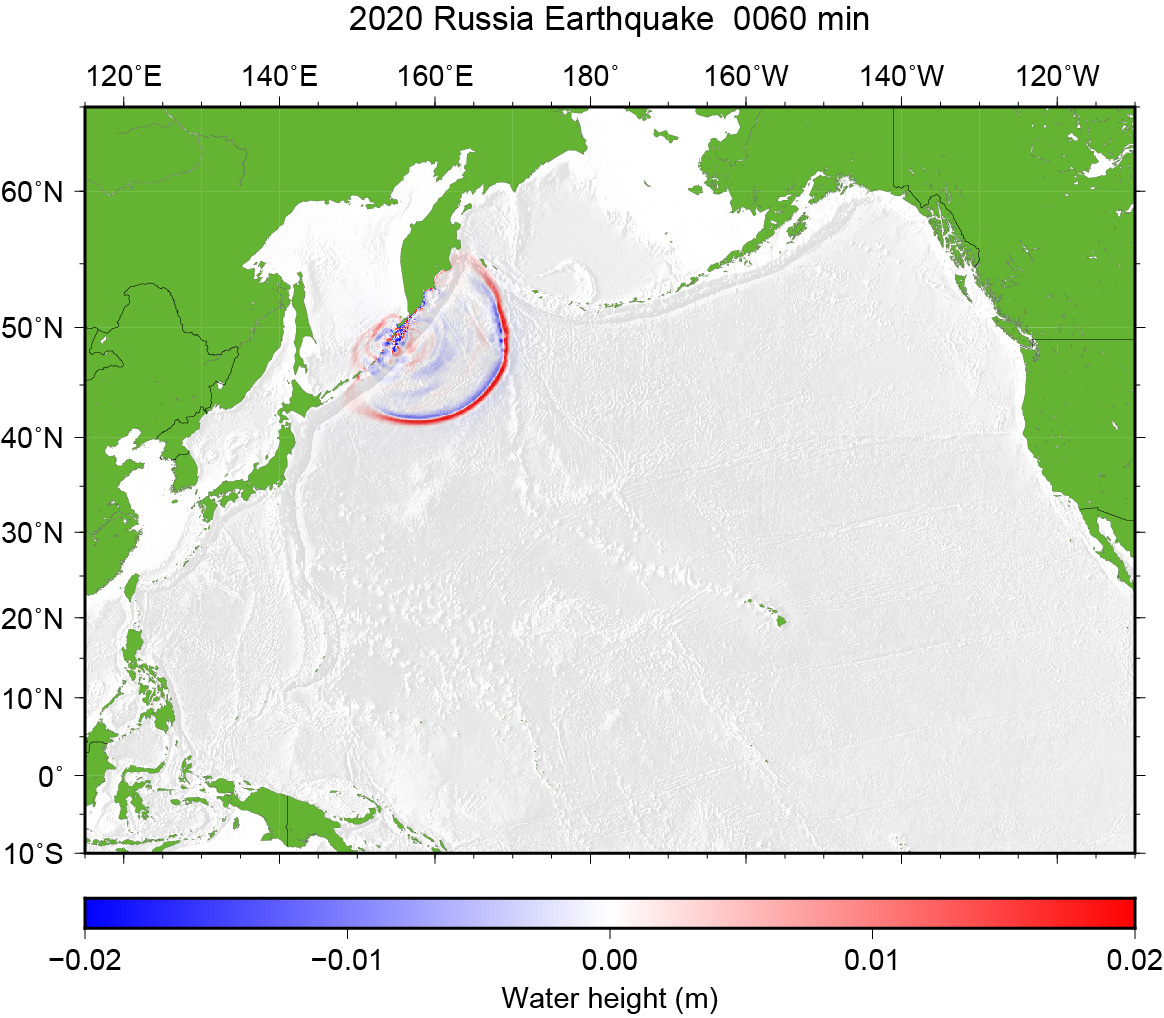
Fig.3 Tsunami propagation

Fig.3 Tsunami Propagation (Click to start animation)
The red color means that the water surface is higher than normal sea level, while the blue means lower.


 

by Yushiro Fujii (IISEE, BRI) and Kenji Satake (ERI, Univ. of Tokyo)
 
 
References
Okada, Y. (1985), Surface Deformation Due to Shear and Tensile Faults in a Half-Space, Bull. Seismol. Soc. Am., 75, 1135-1154.
Satake, K. (1995), Linear and Nonlinear Computations of the 1992 Nicaragua Earthquake Tsunami, Pure and Appl. Geophys., 144, 455-470.
Watada, S., S. Kusumoto and K. Satake (2014), Traveltime delay and initial phase reversal of distant tsunamis coupled with the self-gravitating elastic Earth, J. Geophys. Res., 119, 4287-4310.


Last Updated on 2020/3/25